 Back to Widom research group page
Back to Widom research group page
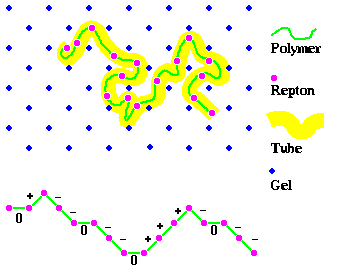
Gel electrophoresis separates charged polymers, such as sections of DNA, according to their length. Applied electric fields exert a uniform force per unit length parallel to the field. The gel contains pores and fibers which tend to entrap the long polymers within tubes, impeding their drift. Significant motion of the polymer occurs by the transport of stored length through the tube, a process known as reptation introduced by de Gennes. As a result of entanglement of the polymer in the gel, the polymer develops a complicated dependence of drift velocity on electric field E and total polymer length L=P N. We denote the polymer persistence length (typically of order 150-300 base pairs for DNA) as P, and call N the ``chain length'' of the polymer.
In the limit of weak field, the velocity is proportional to the
electric force through the Nernst-Einstein relation
v = (D/kT)(q N E)
Here (q N E) is the electric force on the polymer, with q the
charge within a persistence length. The diffusion constant D depends
on the chain length according to the prediction of de Gennes
D ~ N^{-2}.
Comparing the above equations, the weak field drift velocity varies
inversely with the chain length. Short chains travel more quickly
than long chains, and chains may be sorted according to length based
on their travel time across the gel, or their travel distance within a
given time.
Loss of length resolution, a serious impediment to electrophoresis as a separation tool, occurs for any polymer above a certain length. For DNA typical limits are of order 50 Kbp, with chain length N of order 150-300. Increasing these limits requires reducing the electric field, thus increasing the run time, or using more delicate gels. Pulsed-field and other techniques push the threshold length out yet further, reaching DNA lengths of order 10^7 bp, with chain length N of order 10^5. From a mathematical point of view, loss of length resolution implies a breakdown of the Nernst-Einstein relation between drift velocity and zero-field diffusion constant. Understanding this problem requires further investigation of the functional form of the drift velocity v(N,E).
Click here for a postscript copy of "Repton model of gel electrophoresis in the long chain limit" by M. Widom and I. Al-Lehyani (submitted to Physica A, 1997).